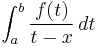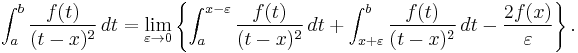Hadamard regularization
| Renormalization and regularization |
|---|
|
Renormalization
|
|
Dimensional regularization
Pauli–Villars regularization Lattice regularization Zeta function regularization Causal perturbation theory Hadamard regularization Point-splitting regularization |
In mathematics, Hadamard regularization (also called Hadamard finite part or Hadamard's partie finie) is a method of regularizing divergent integrals by dropping some divergent terms and keeping the finite part, introduced by Hadamard (1923, book III, chapter I, 1932). Riesz (1938, 1949) showed that this can be interpreted as taking the meromorphic continuation of a convergent integral.
If the Cauchy principal value integral
exists, then the Hadamard finite part integral can be defined as
Also it can be calculated from the definition
References
- Blanchet, Luc; Faye, Guillaume (2000), "Hadamard regularization", Journal of Mathematical Physics 41 (11): 7675–7714, doi:10.1063/1.1308506, ISSN 0022-2488, MR1788597
- Hadamard, Jacques (1923), Lectures on Cauchy's problem in linear partial differential equations, Dover Phoenix editions, Dover Publications, New York, ISBN 978-0-486-49549-1, JFM 49.0725.04, MR0051411, http://books.google.com/books?id=B25O-x21uqkC
- Hadamard, J. (1932) (in French), Le problème de Cauchy et les équations aux dérivées partielles linéaires hyperboliques, Paris: Hermann & Cie., pp. 542, Zbl 0006.20501
- Riesz, Marcel (1938), "Intégrales de Riemann-Liouville et potentiels." (in French), Acta Litt. Sci. Szeged 9: 1–42, Zbl 0018.40704
- Riesz, Marcel (1949), "L'intégrale de Riemann-Liouville et le problème de Cauchy", Acta Mathematica 81: 1–223, doi:10.1007/BF02395016, ISSN 0001-5962, MR0030102